Why Birds Don?

+ BY DON BOROUGHS
+ ILLUSTRATIONS BY STUART BRADFORD

Eva Kanso has questions about the physical world. The University of Southern California mechanical engineering professor wants to know why fish are shaped the way they are, why they flap their tails in a particular motion, and how to design a better robotic fish. But Kanso is also an applied mathematician, so she doesn’t need to get wet. “Applied mathematics is the tool I use to answer whatever questions I have,” Kanso says. With a speed and precision no experimentalist can match, she adjusts her mathematical models to change the weight of a fish, the shape of its fins, and the frequency of its undulations. Instantly she can observe the vortices in the fish’s wake displayed on her computer monitor. “Mathematically,” she brags, “you can play God.”

If mathematics is the language of engineering, then the engineers who use applied mathematics are master linguists. Applied mathematicians develop new mathematical models or adapt existing models to address engineering problems. A 100-fold increase in computing power over the past decade has multiplied the power of applied math at the same time that engineers are tackling more complex, interdisciplinary problems that resist solutions by trial-and-error experimentation alone. “Engineering without mathematics and applied mathematics is like sailing without a compass,” says Mathieu Desbrun, professor of computing and mathematical sciences in Caltech’s Division of Engineering and Applied Science. “You can do it, but it’s very hard.”

Not that applied mathematics obviates the need for experimentation. The most traditional form of collaboration between an experimentalist engineer and an applied mathematician sees the mathematical model suggesting new directions for experiments while the results of lab work in turn help refine the model. “You iterate back and forth between experiment and theory until you get a coherent story; that’s when you’ve made progress,” says John Bush, an applied mathematician who directs the Fluids Lab at the Massachusetts Institute of Technology.

A disproportionate number of academics with applied mathematics expertise — including Kanso, Desbrun, and Bush — began their education outside the United States. Guillermo Sapiro, a Uruguayan who studied in Israel, notes that the undergraduate electrical engineering curriculum at the Technion in Haifa required him to take a semester of Fourier analysis and a semester of complex theory before launching into signals and systems. His students at the University of Minnesota, by contrast, have all three of those subjects crammed into a one-semester signals and systems course. Hossein Haj-Hariri, chair of mechanical and aerospace engineering at the University of Virginia, laments that most American grad students today are too far behind in mathematics to launch into a computational field such as hydroacoustics: “By the time they learn the math and are ready to produce something, four years have passed and it’s time to graduate.”

Still, American universities are well populated with engineers from near and far who undertake innovative research using applied mathematics, either through their own expertise or in collaboration with mathematicians. Some of the hottest fields in engineering, from image processing to data mining and from bio-inspired design to complex systems, are the very same fields where applied mathematics plays a key role in advancing research. When applied mathematics and engineering join forces, one plus one equals a sum much greater than two.

Sending Cars to School

Complex network systems such as the Internet or the smart grid depend on close coordination between engineers and applied mathematicians. But the networks that inspired Reza Olfati-Saber were the schools of fish he followed while snorkeling off the shores of Hawaii. The electrical engineer and control theorist developed a series of algorithms for schooling and flocking that in five years have been cited more than 2,000 times and confirmed by numerous biological studies.

Today, half a world away in New Hampshire, Olfati-Saber watches a miniature school of robots in a basement at Dartmouth’s Thayer School of Engineering. He is convinced that the same mathematical rules that coordinate the movements of fish and flocks can save lives and eliminate traffic jams by coordinating vehicles’ movements. His model explains that thousands of birds manage to move together without ever colliding because each bird is monitoring its distance from just five or six birds around it. Likewise, he envisions a traffic system of vehicles that negotiate position with their nearest neighbors — without driver intervention. To reach that goal, Olfati-Saber first hopes to raise $10 million to test his intelligent-transportation system with several unmanned cars on mocked-up roads.

When Olfati-Saber compares his work with earlier, trial-and-error research on flocking, he sees proof of applied mathematics’ superiority. In 1986, simulation software for flocking was developed using three intuitive rules. The program has been used to animate video games and films, and even won a technical Oscar, but no engineering system or mathematical model was ever derived from its ad hoc rules. “No matter how many times you do simulations on computers, they were unable to come up with a set of rules that guarantee no collisions,” says Olfati-Saber. His birds, fish, and cars operate according to algorithms based on tools from graph theory, control theory, applied mechanics, and nonlinear dynamics. And under normal circumstances, they never crash. The engineer laughed when he finally learned how Hollywood animators avoid on-screen collisions: They simply take the wayward bird, bat, or fish and delete it.

Many challenges in the rapidly growing field of image processing involve helping consumers and corporations visually filter billions of images now on the Internet. Sapiro’s challenge is to save lives. The Minnesota professor works on a National Institutes of Health team seeking an AIDS vaccine. A key step in that quest is to understand the shape of a spike, made up of just three molecules, that HIV uses to attach itself to cells. The NIH has one of the best medical electron microscope facilities in the world, but to avoid melting and deforming the frozen virus samples, technicians aim miserly beams of electrons at the specimens.

The resulting images are “blobs,” Sapiro says, “like a picture taken in a dark room with no flash.” Yet with algorithms using Fourier analysis and matrix analysis, Sapiro and his students have coaxed detailed, even beautiful images from the raw files. The mathematical alchemy depends on computations that compare details in multiple images. To form a three-dimensional model, the electron microscope captures 60 to 70 images from different angles, and where noise blots out detail in one image, the algorithm finds detail in another. Unlike commercial software that might retouch scratches on an old photograph by averaging tones around the scratch, Sapiro’s algorithms fill in only actual details. “I should not be inventing information,” he says. “In medical imaging, that’s dangerous.”

There can be no doubt that the NIH values this work. The institute has lured two of Sapiro’s electrical-engineering protégés — Ph.D. student Alberto Bartesaghi and Oleg Kuybeda, a post-doc — from Minnesota to its Bethesda, Md., laboratories. And Sapiro takes pleasure in applying mathematics to a problem that is “exciting, real, and extremely tough at the same time.” The challenge is its own reward.

A Ray of Flight

Both engineering and applied mathematics are converging on the living world as a final frontier. “Biology is the last field to really become quantified in a serious sense,” notes James Crowley, executive director of the Society for Industrial and Applied Mathematics. Within universities, one sign of the revolution is the fact that Harvard and Georgia Tech have both opened institutes dedicated to biologically inspired engineering in recent years.

One of the most ambitious projects in the field spans the engineering schools of Princeton, UCLA, and the University of Virginia in a $6.5 million effort to unlock the secrets of stingray and manta ray propulsion for the U.S. Navy. Principal investigator Hilary Bart-Smith, an associate professor of mechanical and aerospace engineering at U.Va., says that the manta ray is both efficient and maneuverable. “It could be an excellent species to use as the model for the next generation of autonomous underwater vehicles,” she argues, “and it’s pretty darn cool, too.”

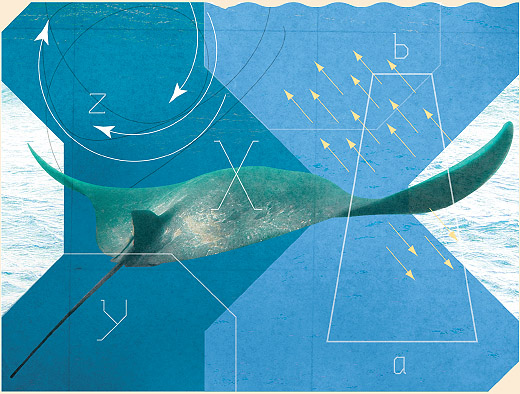
The manta ray could be a model for autonomous underwater vehicles.

But rays are also complicated, swimming by a combination of wing-flapping and undulating ripples. “We want to understand the interaction of fluids and this structure of muscle, cartilage, and neurons; the thing that ties all these together is mathematics,” says Haj-Hariri, one of the project’s applied mathematicians.

Bart-Smith points out the advantages of mathematical modeling by noting that one of her graduate students has spent months testing a one-wing structure in a single flapping motion, varying the frequency alone. In contrast, Haj-Hariri’s model allows him to adjust the shape, kinematics, and frequency simultaneously, measuring the results in near real time. “You can’t ignore the experiments, but once you verify that mathematically you are capturing what’s happening in reality, then you can really crunch numbers,” says Bart-Smith. “The power of applied math is that it gives you the opportunity to explore all of the design space, rather than just parts of the design space.”

The models are also leading the team beyond manta rays and toward a grand theory that will help explain the locomotion of all fish and swimming mammals. Haj-Hariri hints that the team’s research points to a mathematical connection among the natural frequencies of the biomechanical design of each creature and the frequencies of both their flapping and their brain neurons. It seems that nature herself is an engineer with an inordinate fondness for applied mathematics.

Don Boroughs is a freelance writer based in South Africa.